ATOMIC STRUCTURE NOTES

Hydrogenic Atoms

Orbital Angular Momentum & Electron Spin

Quantum Numbers

Principal Quantum Number - Quantized Energy

Orbital Angular Momentum, l - Quantized Orbital Angular Momentum

Magnetic Quantum Number, m_l - Quantized Orientation of angular momentum.

The allowed energies are determined by n, and for a hydrogenic atom of atomic number Z:

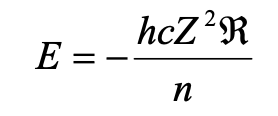
The zero energy is when electron and nucleus are widely separated. 1/n² relationship for E indicates that the energy levels converge at high (less negative) energies. Zero energy = ionisation.

Electron Spin -

2 more Quantum Numbers are needed to define the state of an electron (spin).

s = spin angular momentum and always has a value of ¹/₂.

ms = spin magnetic quantum number, and is either +¹/₂ or -¹/₂.

These Quantum Numbers specify the orientation of the spin with respect to a chosen axis.

Spin-up and Spin-down (α and β).

Shapes and Energies of Atomic Orbitals

Radial Shapes of Hydrogenic Orbitals

A Hydrogenic orbital can be written as the product of a function R of the radius and a function Y of the angular coordinates. R determines variation with distance. Y expresses the shape of the orbital.

Where the radial wavefunction passes through zero is a radial node. Where the angular wavefunction passes thru zero is an angular node or nodal plane.

1s (n=1, l=0, m_l=0) decays exponentially with distance from the nucleus and never passes thru zero.

An orbital with Quantum Numbers n and l in general has n-l-1 radial nodes. Hence 2s (n=2, l=0 m_l=0) passes thru zero once (one radial node).

Multi-electron Atoms

Penetration & Screening

Shielding

An electron is assumed to experience an effective nuclear charge (Z_eff) dependent on radius (location), n and l (differing radial distribution functions). The reduction of the true nuclear charge to the effective nuclear charge is called shielding. Z_eff = Z - σ.

The closer to the nucleus that an electron can penetrate in an atom, the closer the value of Z_eff to Z, because the electron is repelled less by other electrons. For example, a 2s electron can penetrate, while a 2p electron is not as effective at this because it has a nodal plane through the nucleus. Therefore, it is more shielded from the nucleus by the electrons of the core. We can conclude that a 2s electron has lower energy (more bound) than a 2p, so for Lithium the ground state configuration must be 1s²2s¹. It usually follows that: ns > np > nd > nf

Radial Distribution Functions

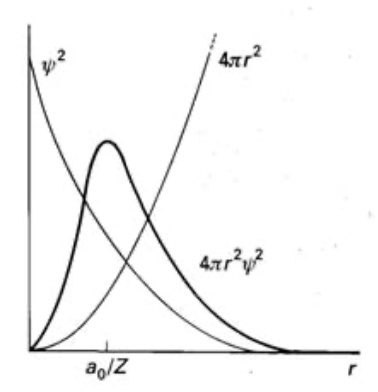
(for 1s orbital)

In general, the distance at which the electron is most likely to be found decreases with nuclear charge. The distance also increases as n increases since the higher the energy, the more likely the electron is to be further away. Maximum (most likely) position occurs at a₀/Z.

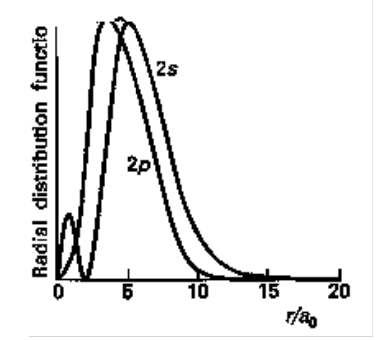
Although 2p is on average closer, the 2s has a higher probability of being close to the nucleus because of the inner maximum.

Periodicity

Ionic Radii

Metallic Radius = half the distance between nuclei of neighbouring atoms.

Covalent Radius = half the inter-nuclear separation of neighbouring atoms of the same element.

General Periodic Trends - descending a group atomic radii increase, and with s & p blocks they decrease from left to right across period.

Period 6 is different, due to lanthanide contraction. The 4f orbitals are being occupied by the lanthanides, and these have poor shielding properties. The repulsions being added on crossing the f-block fail to compensate for the increasing nuclear charge, so all the electrons are drawn inwards (Z_eff increases).

Similar reasoning behind the small increase in radius from Al to Ga - d-block with poor shielding.

Increase in radius of an anion is due to increased electron-electron repulsions. Smaller radius of cations is due to less repulsion and also that there is a less in valence electrons (leaving a more compact core).

Ionization Energy

Follows pattern of Z_eff. Correlate with atomic radii - small radius = high E_I.

Electron Affinity

Electron-gain enthalpy - ∆_egH is the standard enthalpy change per mole of atoms when a gas-phase atom gains an electron. Can be exothermic or endothermic.

A(g) + e^-(g) --> A^-(g)

E_a = E(A,g) - E(A^-,g)

A positive electron affinity indicates that the ion has a lower (more -ve), and so more favourable energy than the neutral atom. Its value is determined by the energy of the lowest unfilled orbital of the ground-state atom. An element has a high electron affinity if the additional electron can enter a shell where it experiences a strong effective nuclear charge. This is the case for elements in the top right of the periodic table.